Complex Numbers

CalculatorCrave complex numbers calculator allows you to perform calculations involving complex numbers with ease. Simply input the real and imaginary parts of the complex numbers you want to add, subtract, multiply, or divide, and watch as the calculator provides you with the precise result. Perfect tool for students and anyone working with complex numbers in their calculations.

What are complex numbers?

Complex numbers are a fundamental concept in mathematics that are essential in various fields such as physics, engineering, and computer science. They are expressed in the form a + bi, where "a" and "b" are real numbers and "i" is the imaginary unit (√-1). These numbers extend the real numbers by introducing the imaginary unit, allowing for the solution of equations that have no real solutions.

Addition, subtraction, multiplication and division of complex numbers

Complex number operations involve addition, subtraction, multiplication, and division. Each operation follows specific rules that must be adhered to for accurate results.

1. Addition:
When adding complex numbers, the real parts and imaginary parts are added separately.

For example, (4 + 2i) + (7 - 3i)

= (4 + 7) + (2i - 3i)

= 11 - i.

2. Subtraction:
Similarly, when subtracting complex numbers, the real parts and imaginary parts are subtracted separately.

For example, (4 + 2i) - (7 - 3i)

= (4 - 7) + (2i - (-3i))

= -3 + 5i.

3. Multiplication:
To multiply complex numbers, we use the distributive property and apply the rules of multiplying imaginary units.

For example, (4 + 2i) * (3 - i)

= 4*3 + 4*(-i) + 2i*3 + 2i*(-i)

= 12 - 4i + 6i - 2i^2

= 14 + 2i.

4. Division:
Dividing complex numbers involves rationalizing the denominator by multiplying by the conjugate of the denominator.

For example, (4 + 2i) / (3 - i)

= ((4 + 2i)*(3 + i)) / ((3 - i)*(3 + i))

= (12 + 4i + 6i + 2i^2) / (9 + 3i - 3i - i^2)

= (14 + 10i) / 10 = 1.4 + i.

The Argand diagram

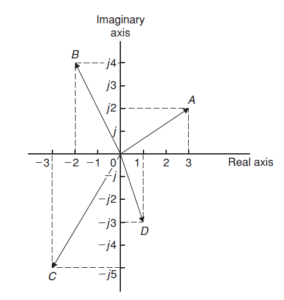
An Argand diagram is a graphical representation used to depict complex numbers in the form of a plane. In an Argand diagram, the horizontal axis represents the real part of the complex number, while the vertical axis represents the imaginary part. This graphical representation was developed by Jean-Robert Argand in the early 19th century and has since become a valuable tool in understanding the properties and operations involving complex numbers.

One of the key operations involving complex numbers represented in an Argand diagram is addition. When adding two complex numbers, you simply add their real parts and their imaginary parts separately. This can be visualized in the Argand diagram by moving from the initial complex number to the complex number being added, then drawing a vector from the initial complex number to the resultant complex number.

Worked example

For example, let's consider the complex numbers z1 = 3 + 2i and z2 = 1 - 4i. In the Argand diagram, you would plot z1 as a point at (3, 2) and z2 as a point at (1, -4). Adding these two complex numbers would involve drawing a vector from z1 to z2, resulting in a new complex number z3 = 4 - 2i. This can be visually represented in the Argand diagram as the vector from (3, 2) to (4, -2).

These operations are essential in solving complex mathematical problems, especially in applications involving electrical engineering, signal processing, and quantum mechanics. Understanding how to perform operations with complex numbers will enhance problem-solving skills and mathematical proficiency.